Match each quadratic equation with its solution set – Matching quadratic equations with their solution sets is a fundamental skill in algebra. It involves finding the values of the variable that make the equation true. This guide will provide an overview of quadratic equations, methods for solving them, and techniques for matching them with their solution sets.
Quadratic equations are second-degree polynomial equations of the form ax^2 + bx + c = 0, where a, b, and c are constants and a is not equal to zero. The solutions to a quadratic equation are the values of x that satisfy the equation.
Overview of Quadratic Equations: Match Each Quadratic Equation With Its Solution Set
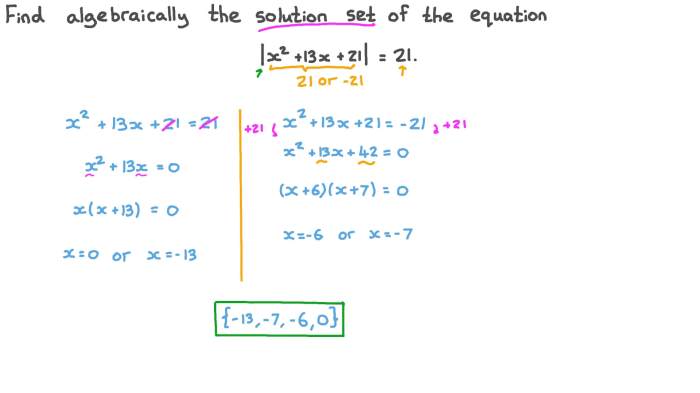
A quadratic equation is a polynomial equation of degree two, written in the standard form ax 2+ bx + c = 0, where a, b, and c are real numbers and a ≠ 0. The solutions to a quadratic equation are the values of x that make the equation true.
Quadratic equations are important in many areas of mathematics and science, and they have a variety of applications in the real world.
Methods for Solving Quadratic Equations
Factoring Method
The factoring method involves finding two numbers that add up to b and multiply to ac. These numbers can then be used to factor the quadratic equation into two binomials, which can then be set equal to zero and solved for x.
Quadratic Formula Method
The quadratic formula is a general formula that can be used to solve any quadratic equation. The formula is x = (-b ± √(b 2– 4ac)) / 2a.
Completing the Square Method, Match each quadratic equation with its solution set
Completing the square is a method that involves adding and subtracting a constant term to the quadratic equation so that it can be written in the form (x + h) 2= k. This equation can then be solved for x.
Matching Quadratic Equations with Solution Sets
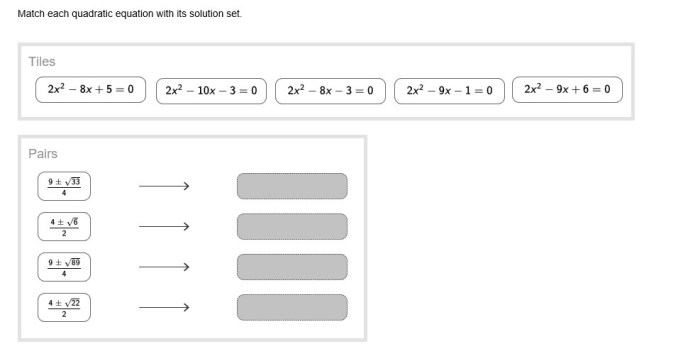
To match a quadratic equation with its solution set, we can use either the factoring method or the quadratic formula method to solve the equation. The solution set will be the set of all values of x that make the equation true.
| Quadratic Equation | Solution Set |
|---|---|
x2
|
1, 3 |
| x2+ 2x + 1 = 0 | -1 |
x2
|
2, 3 |
Examples and Non-Examples
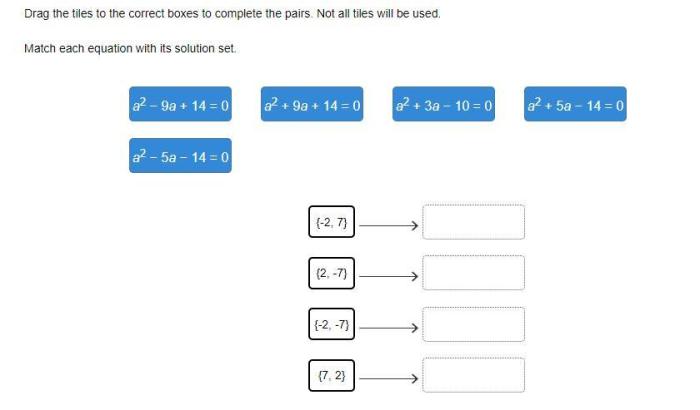
Examples
The following are examples of quadratic equations:
- x 2– 4x + 3 = 0
- x 2+ 2x + 1 = 0
- x 2– 5x + 6 = 0
Non-Examples
The following are not examples of quadratic equations:
- x 3– 4x + 3 = 0
- x 2+ 2x = 0
- x – 5 = 0
Applications of Quadratic Equations
Quadratic equations have a variety of applications in the real world. Some examples include:
- Projectile motion
- Circuit analysis
- Economics
Helpful Answers
What is the standard form of a quadratic equation?
The standard form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants and a is not equal to zero.
What are the methods for solving quadratic equations?
The three main methods for solving quadratic equations are factoring, the quadratic formula, and completing the square.
How do I match a quadratic equation with its solution set?
To match a quadratic equation with its solution set, solve the equation using one of the methods mentioned above and find the values of x that satisfy the equation.